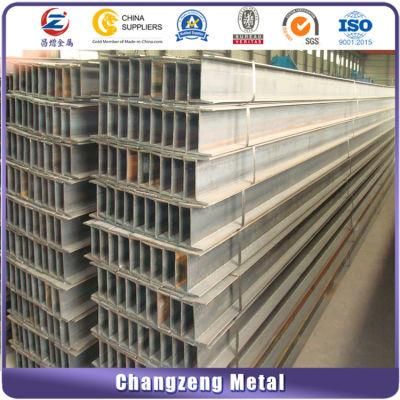
H Beam Double-T Steel (CZ-H79)
Shanghai Changzeng Metal Co., Ltd.- Function:Structure Building
- Thickness:5-28mm
- Standard:GB, ASTM, AISI
- Grade:Structural Steel
- Flange Width:50-400mm
- Web Thickness:6.5-70mm
Base Info
- Application:Steel Workshop, Steel Structure Platform, Steel Walkway and Floor, Steel Structure Bridge
- Residential Wall Structure:Connector
- Carbon Structural Steel:Q235
- Type of Steel For Building Structure:High-Strength Steel
- Member:Steel Beam
- Connection Form:Welded Connection
- Forming:Hot-Rolled Steel
- Trademark:Carbon Structural Steel, CZ
- Type:H-Section Steel
- Length:12m or Custom
- Item Type:Steel H Beam
- Technique:Hot Rolled
- Surface Treatment:Galvanized, Coated or Custom
- Model NO.:CZ-H79
- Transport Package:1. Big Od, Bulk Small Od: Packed by Steel Strip
- Specification:GB, ASTM, AISI
- Origin:Shanghai
- Production Capacity:5000 Tons, Month, 15000 Tons, Season
Description
Standard Sectional DimensionSection Unit Weight Informative Reference Area Geometrical Radius of Modulus of Nominal H X B t1 t2 r A moment of inertia Gyration of area Section Dimensional
mm mm mm mm mm cm2 kg/m kg/12m 1x cm4 1ycm4 ix cm iy cm Zx cm3 Zy cm3 100 x 100 100 x100 6 8 10 21.90 17.20 206 383 134 4.18 2.47 76.50 26.7 125 x 125 125 x125 6.5 9 10 30.31 23.80 286 847 293 5.29 3.11 136 47.00 150 x150 150 x150 7 10 11 40.14 31.50 378 1,640 563 6.39 3.75 219.00 75.10 175 x175 175 x175 7.5 11 12 51.21 40.20 482 2,880 984 7.50 4.38 330.00 112.00 200 x200 200 x200 8 12 13 63.53 49.90 599 4,720 1,600 8.62 5.02 472.00 160.00 250 x250 250 x250 9 14 16 92.18 72.40 869 10,800 3,650 10.80 6.29 867.00 292.00 300 x300 300 x300 10 15 18 119.80 94.00 1128 20,400 6,750 13.10 7.51 1,360.00 450.00 350 x350 350 x350 12 19 20 173.9 137.00 1644 40,300 13,600 15.20 8.84 2,300.00 776.00
Packaging & Delivery:Packaging details1.Big order: in bulk 2.Small order: packed by steel strips 3.woven cloth with 7 slats 4.according to the requirements of customers 5. 20ft container can load 25 tons, 40ft container can load 26 tons. Delivery 3-15 working days after received the deposit or L/C.
Description:
Beams are designed to support mainly vertical loads and are small in cross-section compared with their span. A beam is a structural member which spans horizontally between supports and carries loads which act at right angles to the length of the beam.
The detailed behaviour of a beam is complex and exact analysis requires considerable mathematical sophistication. However, the vast majority of beams can be designed using engineering beam theory. This is based on a number of simplifying assumptions. Engineering beam theory identifies two types of internal force - bending moments and shear forces. the behaviour of any beam is characterised by the magnitude and distribution of these forces.
Beams deflect when loaded and this must be limited to avoid damage and distress. Beam deflections can be calculated and depend on the modulus of elasticity of the material, and the moment of inertia of the cross-section. In general, the calculation of deflection is not straight forward. However, algebraic expressions are tabulated for many standard cases. The deflection of a particular beam is inversely proportional to:
1).modulus of elasticity
2).moment of inertia
Payment term:
Contact:
mm
Packaging & Delivery:Packaging details
Description:
Beams are designed to support mainly vertical loads and are small in cross-section compared with their span. A beam is a structural member which spans horizontally between supports and carries loads which act at right angles to the length of the beam.
The detailed behaviour of a beam is complex and exact analysis requires considerable mathematical sophistication. However, the vast majority of beams can be designed using engineering beam theory. This is based on a number of simplifying assumptions. Engineering beam theory identifies two types of internal force - bending moments and shear forces. the behaviour of any beam is characterised by the magnitude and distribution of these forces.
Beams deflect when loaded and this must be limited to avoid damage and distress. Beam deflections can be calculated and depend on the modulus of elasticity of the material, and the moment of inertia of the cross-section. In general, the calculation of deflection is not straight forward. However, algebraic expressions are tabulated for many standard cases. The deflection of a particular beam is inversely proportional to:
1).modulus of elasticity
2).moment of inertia
Payment term:
| 1 | 100% T/T in advance. |
| 2 | 30% deposit by T/T;70% balance pay against copy of B/L; |
| 3 | L/C. |
Contact:
| Contact | Anna |
| Fax | 021-66866895 |
| Tel | +8618715010658 |
